Continuous time Carrying Charges Suppose That a Continuous time Compounding Framework
Continuous compounding is the mathematical limit reached by compound interest when it's calculated and reinvested into an account balance over a theoretically endless number of periods. Put simply, the account balance continually earns interest, and that interest gets added to the balance, which then also earns interest and it continues to grow. The concept of continuously compounding is important in finance though it is not possible in practice. The majority of the interest is compounded on a monthly, quarterly, or semiannual basis, so it is an extreme case of compounding. This formula determines the interest that is earned on an amount by constantly compounding it to a theoretically infinite amount of compounding periods. Put simply, it takes compounding to the furthest theoretical limit. Interest will be calculated assuming that there is a constant compounding over an infinite number of periods instead of being calculated on a finite period such as yearly or monthly. The constant compounding formula is derived from the future value of an interest-bearing investment formula, which is more commonly referred to as the compound interest formula: Without getting too mathematical, the continuous compounding formula is much simpler because with finite compounding you have to raise the value to a large exponent, which is messier than the cleaner continuous formula. Jeremy is looking at investment opportunities and has $15,000 to invest, with an expected interest of 14% over the next year. We can use the finite and continuous compounding formulas above to find the ending value of the investment: As you can see in these examples, continuous compounding is only marginally more than daily compounding. Even though we're using a theoretically infinite number of compounding, the final amount is not much more because the effect of each compound becomes smaller each time. When calculating continuous compounding, the below points are worth bearing in mind as a quick recap of what it is, why it's used, and how to use it: You can use the continuous compounding calculator below to work out your future value and compare it with finite compounding periods. Continuous compounding is the mathematical limit reached by compound interest when it's calculated and reinvested over unlimited periods. In other words, it takes compounding to the furthest theoretical limit. The formula to calculate continuous compounding is: FV = PV × eit where: In theory, continuous compounding means that an account balance will grow infinitely over time, as compound interest is reinvested and earns interest on top of interest. Theoretically, yes, banks could use continuous compounding. However, in practice, it's impossible to have an infinite number of periods. This is because there are practical limitations to how often compound interest can be calculated and reinvested. One example of continuous compounding in action is an account that earns interest at a rate of 14% per year, compounded monthly. The balance continually earns interest, which is added to the balance, and because there are 12 months in a year, the account balance increases by 1.17% each month. Over time, this leads to a significantly larger balance. Continuous Compounding Formula
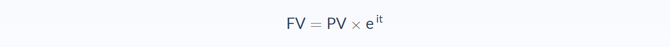
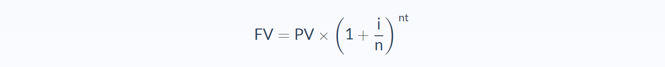
Continuous Compounding Example

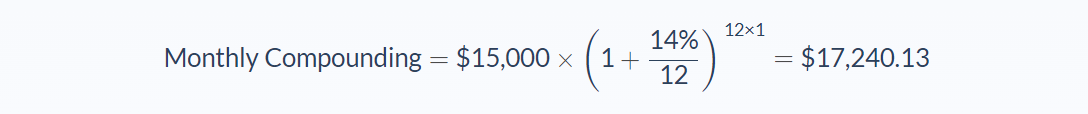

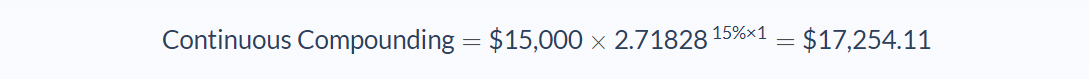
Continuous Compounding Conclusion
Continuous Compounding Calculator
FAQs
1. What is continuous compounding?
2. What is the formula used to calculate continuous compounding?
FV = the future value of the investment
PV = the present value of the investment, or principle
e = Euler's number, the mathematical constant 2.71828
i = the interest rate
t = the time in years 3. What does continuous compounding tell you?
4. Do banks use continuous compounding?
5. What is an example of continuous compounding in action?
ferrellovelinterst.blogspot.com
Source: https://www.carboncollective.co/sustainable-investing/continuous-compounding
0 Response to "Continuous time Carrying Charges Suppose That a Continuous time Compounding Framework"
Post a Comment